Computational Differential Geometry
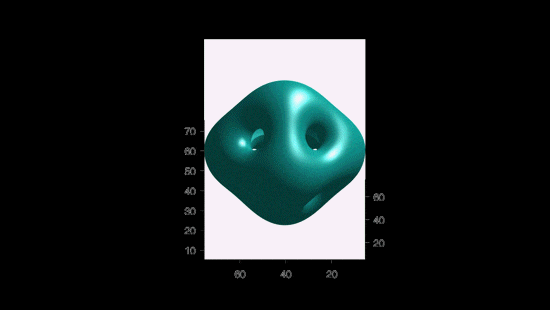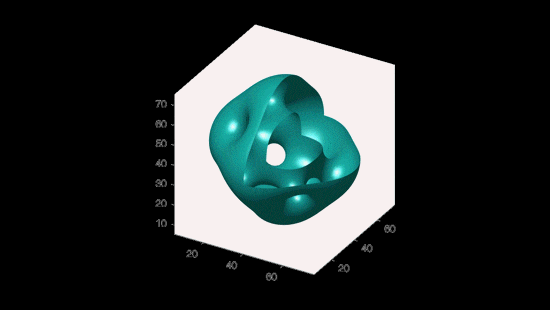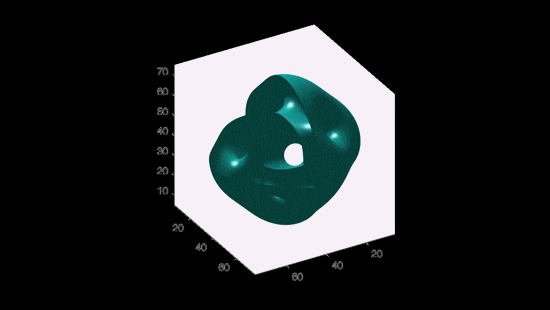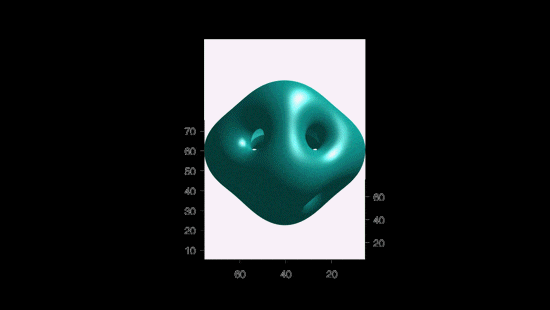
One of the first applications of the Level Set Method was to computing solutions to so-called mean-curvature flow. Mean-curvature flow is where a surface evolves where at every point on the surface the speed of point is equal to the local mean curvature in the direction normal to the surface. For example, a sphere has constant mean curvature and so the resulting flow is a sphere that shrinks with speed equal to the inverse of its radius. A natural application is a simple model for soap films where the curvature represents the force due to surface tension. In fact, the method was used to compute minimal surfaces, i.e. equilibrium shapes for surfaces evolving under curvature flow with fixed boundary. The image at the left represents the shape of a surface that, if evolved under curvature flow, would shrink self-similarly like the sphere example.
Chopp was an early pioneer in the use of the Level Set Method in the context of differential geometry. The first application to minimal surfaces led to key insights into the Level Set Method that led to its being useful for a wider array of applications. Other applications include flow under Gaussian curvature, self-similar surfaces for curvature flow, and curvature flow in hyperbolic space. The techniques are useful for theoreticians to develop a better insight into special cases that are not easy to visualize.
Computational Differential Geometry Papers
Geometry Articles
| Author(s) | Title/Journal | Year |
|---|---|---|
| M. Torres, D. L. Chopp, and T. Walsh | Level set methods to compute minimal surfaces in a medium with exclusions (voids). Interfaces and Free Boundaries, 7(2) | 2005 |
| D. L. Chopp and J. A. Velling | Foliations of hyperbolic space by constant mean curvature surfaces sharing ideal boundary. Journal of Experimental Mathematics, 12(3):339-350 | 2003 |
| D. L. Chopp and J. A. Sethian | Motion by intrinsic Laplacian of curvature. Interfaces and Free Boundaries, 1(1):107-123 | 1999 |
| D. L. Chopp, L. C. Evans, and H. Ishii | Waiting time effects for Gauss curvature flows. Indiana University Math Journal, 48(1):311-334 | 1999 |
| S. Angenent, T. Ilmanen, and D.L. Chopp | A computed example of nonuniqueness of mean curvature flow in R3. Communications on Partial Differential Equations, 20(11-12):1937-1958 | 1995 |
| D. L. Chopp | Numerical computation of self-similar solutions for mean curvature flow. Journal of Experimental Mathematics, 3(1):1-15 | 1994 |
| D. L. Chopp and J. A. Sethian | Flow under curvature: Singularity formation, minimal surfaces, and geodesics, Journal of Experimental Mathematics, 2(4):235-255 | 1993 |
| D. L. Chopp | Computing minimal surfaces via level set curvature flow, Journal of Computational Physics, 106(1):77-91 | 1993 |
