The world is full of oscillatory systems, from physics to chemistry to engineering to biology. When two or more oscillators interact, surprising things can happen.
Kuramoto model
The Kuramoto model is a well-studied system in which a set of oscillators undergoes synchronization as the coupling strength is increased. It consists of a N limit-cycle oscillators, arranged in such a way that every oscillator is coupled equally to every other. Each oscillator has its own intrinsic natural frequency, and the coupling is such that the oscillators tend to increase or decrease their frequency to approach the mean phase of the other oscillators.
One remarkable aspect of the model is that the equations governing each oscillator are fully nonlinear, yet the model is exactly solvable.
London's Millennium Bridge
The behavior leading to the infamous wobble of London’s Millennium Bridge in June of 2000 was likely a result of unintentional synchronization of pedestrian footfalls while crossing the bridge. Treating individual walkers as oscillators that push on the bridge side-to-side, my collaborators and I were able to explain many aspects of the instability. We believe that what occurred on opening day was a physical manifestation of the same phenomenon that is at the heart of the Kuramoto model.
For more information, please see “Crowd synchrony on the Millennium Bridge.”

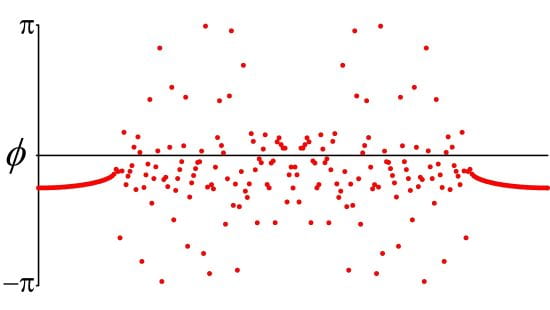
Chimera states
Pattern formation has been a topic of interest for some time, and can occur in a variety of systems. Most mechanisms for the formation of patterns have already been studied, but a new mechanism was discovered in a computer simulation by Yoshiki Kuramoto and Dorjsuren Battogtokh of Kyoto University in 2002. What they found was that a system of completely identical oscillators, with all oscillators coupled in an identical manner to their neighbors, could split into two distinct pieces: one synchronized and the other seemingly random. This astonishing result formed the basis of my work on understanding where this “chimera” state comes from and under what conditions it can exist.
For more information, please see, e.g., “Chimera states for coupled oscillators,” “Chimera states in a ring of nonlocally coupled oscillators,” “Solvable model for chimera states of coupled oscillators,” or “Chimera states: Coexistence of coherence and incoherence in networks of coupled oscillators.”